Introduction
The truncated cube, or truncated hexahedron, is an Archimedean solid.
It has 14 regular faces (6 octagonal and 8 triangular), 36 edges, and 24 vertices.
Products in class
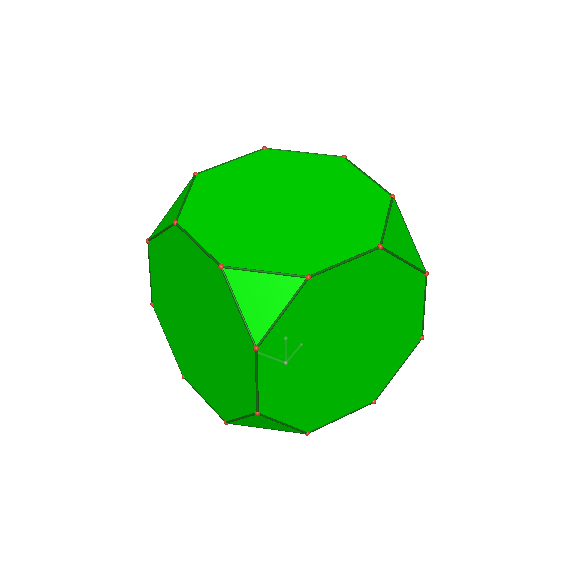
| Small rhombicosidodecahedron | Top |
Introduction
The rhombicosidodecahedron, or small rhombicosidodecahedron, is an Archimedean solid,
one of thirteen convex isogonal nonprismatic solids constructed of two or more types of regular polygon faces.
Products in class

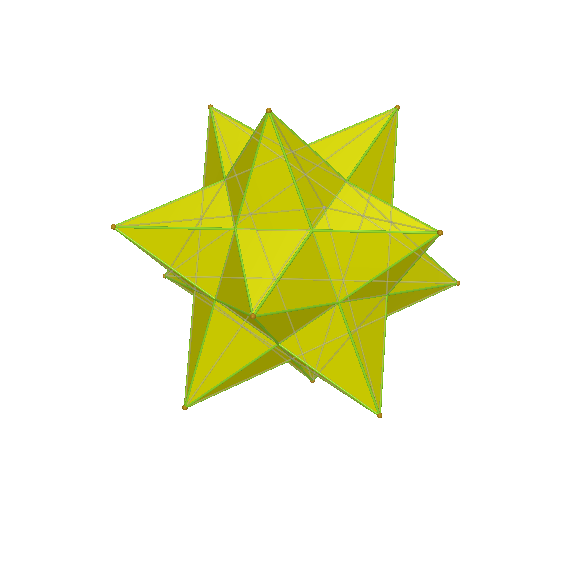
| Small stellated dodecahedron | Top |
Introduction
The small stellated dodecahedron is a Kepler-Poinsot polyhedron,
with Schläfli symbol {5/2,5}. It is one of four nonconvex regular polyhedra.
It is composed of 12 pentagrammic faces, with five pentagrams meeting at each vertex.
Products in class
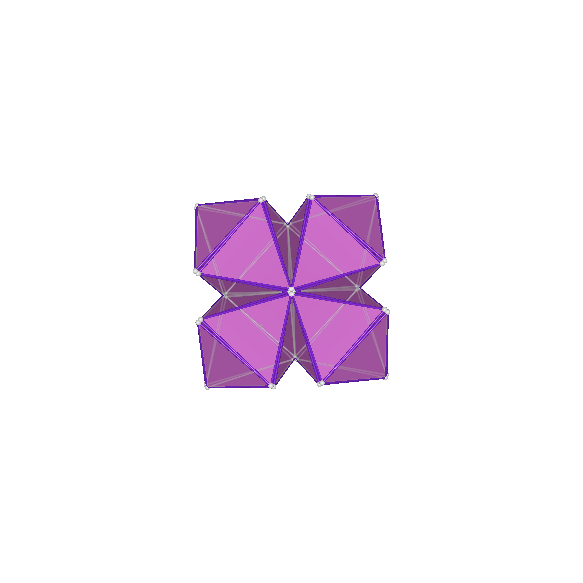
| Brick to form Small stellated dodecahedron | Top |
Introduction
This is an interesting applicaton of Small stellated dodecahedron.
We construct it by bricking.
Products in class

| Great rhombicuboctahedron | Top |
Introduction
The great rhombicuboctahedron is an equilateral zonohedron and the Minkowski sum of three cubes.
It can be combined with cubes and truncated octahedra into a regular space-filling pattern.
Products in class

Introduction
The dodecadodecahedron is a nonconvex uniform polyhedron, indexed as U36.
It is given a Schläfli symbol t1{5/2,5}.
Products in class
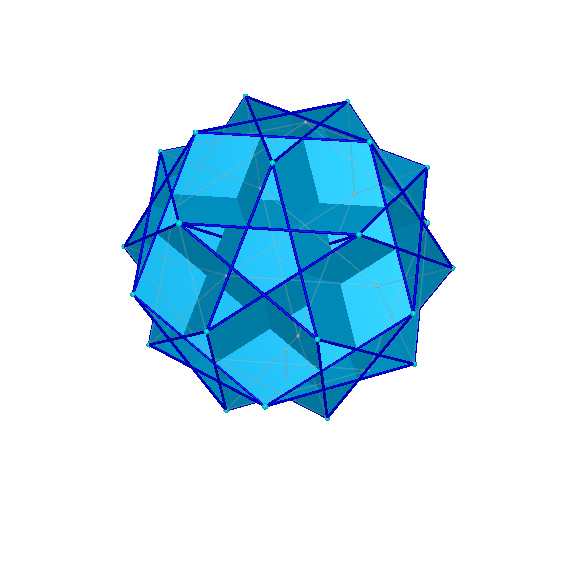
Introduction
The great dodecahedron is a Kepler-Poinsot polyhedron, with Schläfli symbol {5,5/2} .
It is composed of 12 pentagonal faces (six pairs of parallel pentagons),
with five pentagons meeting at each vertex, intersecting each other making a pentagrammic path.
Products in class

Introduction
The truncated octahedron is an Archimedean solid.
It has 14 faces (8 regular hexagonal and 6 square), 36 edges, and 24 vertices.
Products in class
| Octahemioctahedron Dance | Top |
Introduction
This is an interesting applicaton of Octahemioctahedron.
Products in class